12月, 2013 | Improve Society

Ordinary differential equations

Definition of a differential equation

A differential equation is an equation involving derivatives or differentials.

Equations involving only one independent variable are called ordinary differential equations. Equations with two or more independent variables are called partial differential equation.

Order of a differential equation

An equation having a derivative of nth order but no higher is called an nth order differential equation.

Arbitrary constants

An arbitrary constant, often denoted by a letter at the beginning of the alphabet such as A, B, C, c₁, c₂, etc., may assume values independently of the variables involved. For example in $y = x^2 + c_1x + c_2$ , c₁ and c₂ are arbitrary constants.

The relation of $y = Ae^{-4x + B}$ which can be written $y = Ae^Be^{-4x} = Ce^{-4x}$ actually involves only one arbitrary constant. It’s always assumed that the minimum number of constants is present, i.e. the arbitrary constants are essential.

Solution of a differential equation

A solution of a differential equation is a relation between the variables which is free of derivatives and which satisfies the differential equation identically. $y = x^2 + c_1x + c_2$ is a solution of $y'' = 2$ since by substitution the identity 2 = 2.

A general solution of an nth order differential equation is one involving n (essential) arbitrary constants. Since $y = x^2 + c_1x + c_2$ has two arbitrary constants and satisfies the second order differential equation $y'' = 2$ , it is a general solution of $y'' = 2$ .

A particular solution is a solution obtained from the general solution by assigning specific values to the arbitrary constants. $y = x^2 - 3x + 2$ is a particular solution of $y'' = 2$ and is obtained from the general solution $y = x^2 + c_1x + c_2$ by putting c₁ = -3 and c₂ = 2.

A singular solution is a solution which cannot be obtained from the general solution by specifying values of the arbitrary constants. The general solution of $y = xy' - y'^2$ is $y = cx - c^2$ . However, as seen by substitution another solution is $y = x^2/4$ which cannot be obtained from the general solution for any constant c. This second solution is a singular solution.

Differential equation of a family of curves

A general solution of an nth order differential equation has n arbitrary constants (or parameters) and represents geometrically an n parameter family of curves. Conversely a relation with n arbitrary constants (sometimes called a primitive) has associated with it a differential equation of order n (of which it is a general solution) called the differential equation of the family. This differential equation is obtained by differentiating the primitive n times and then eliminating the n arbitrary constants among the n + 1 resulting equations.

微分方程式の定義

　微分方程式とは微分を含む方程式のことです．

　ただ一つの独立変数を含む方程式を常微分方程式と呼びます．2つ以上の独立変数を含む方程式を偏微分方程式と呼びます．

微分方程式の階数

　n次の微分を持つ方程式がそれ以上高階の微分を持たない時，n階微分方程式と言います．

任意定数

　任意定数とは，しばしば1文字で記述され A, B, C, c₁, c₂ などのようにアルファベットで始まりますが，関与する変数とは独立しているのを前提にしています．例えば $y = x^2 + c_1x + c_2$ という関数においては c₁ と c₂ が任意定数です．

　 $y = Ae^{-4x + B}$ の式の関係は $y = Ae^Be^{-4x} = Ce^{-4x}$ と記述され，事実上ただ一つの任意定数を伴っています．定数の最小数が存在することを前提にしています．すなわち任意定数は不可欠です．

微分方程式の解

　微分方程式の解は変数間の関係であり，微分を持たず同一の微分方程式を満たすものを言います． $y = x^2 + c_1x + c_2$ は $y'' = 2$ の解であり，2 = 2 の同一性を置換したものです．

　n 階の微分方程式の一般解は唯一の n 個の（不可欠な）任意定数を伴います． $y = x^2 + c_1x + c_2$ が 2 つの任意定数を有し，2 階の微分方程式 $y'' = 2$ を満たすため，それは $y'' = 2$ の一般解です．

　特殊解は一般解の任意定数に特殊な値を割り付けることで得られます． $y = x^2 - 3x + 2$ は $y'' = 2$ の特殊解であり，一般解 $y = x^2 + c_1x + c_2$ に c₁ = -3 および c₂ = 2 を代入することで得られます．

　単解は任意定数の値を特定しても一般解からは得られません． $y = xy' - y'^2$ の一般解は $y = cx - c^2$ です．しかし別の置換法によって見てみると $y = x^2/4$ はいかなる定数 c によっても一般解からは得られません．この後者が単解です．

曲線族の微分方程式

　n 階の微分方程式の一般解は n 個の任意定数（または変数）をもち，n 変数の曲線族を幾何学的に表しています．逆に n 個の任意定数との関連は（時に原始関数とも呼ばれますが） n 階の微分方程式と関連付けられており（故に一般解なのですが）族の微分方程式と呼ばれます．この微分方程式は原始関数を n 回微分することにより得られ，その結果 n + 1 個の方程式の中で n 個の任意定数は消えます．

Complex numbers

Complex numbers arose in order to solve polynomial equations such as $x^2 + 1 = 0$ or $x^2 + x + 1 = 0$ which are not satisfied by real numbers. It’s assumed that a complex number has the form a + bi where a, b are real numbers and i, called imaginary unit, has the property that i² = -1. Complex numbers are defined as follows.

Addition.
$\displaystyle (a + bi) + (c + di) = (a + c) + (b + d)i$

Subtraction.
$\displaystyle (a + bi) - (c + di) = (a - c) + (b - d)i$

Multiplication.
$\displaystyle (a + bi)\times(c + di) = ac + adi + bci + bdi^2 = (ac - bd) + (ad + bc)i$

Division.
$\displaystyle \frac{a + bi}{c + di} = \frac{a + bi}{c + di}\times\frac{c - di}{c - di} = \frac{ac + bd}{c^2 + d^2} + \frac{bc - ad}{c^2 + d^2}i$

The ordinary rules of algebra has been used except that replaces i² by -1 wherever it occurs. The commutative, associative and distributive laws also apply to complex numbers. It’s called a and b of a + bi the real and imaginary parts, respectively. Two complex numbers are equal if and only if their real and imaginary parts are respectively equal.

A complex number z = x + iy can be considered as a point P with coordinates (x, y) on a rectangular xy plane called in this case the complex plane or Argand diagram. If the line would be constructed from origin O to P and let ρ be the distance OP and φ the angle made by OP with the positive x axis, you could have from Figure

$\displaystyle x = \rho \cos\phi,\ y = \rho\sin\phi,\ \rho = \sqrt{x^2 + y^2}$
and could write the complex number in so-called polar form as
$\displaystyle z = x + iy = \rho(\cos\phi + i\sin\phi) = \rho cis\phi$
It’s often called that ρ the modulus or absolute value of of z and denote it by |z|. The angle φ is called the amplitude or argument of z abbreviated arg z. It could be also written $\rho = \sqrt{z\bar{z}}$ where $\bar{z} = x - iy$ is called the conjugate of $z = x + iy$ .

If you write two complex numbers in polar form as
$\displaystyle z_1 = \rho_1(\cos\phi_1 + i\sin\phi_1),\ z_2 = \rho_2(\cos\phi_2 + i\sin\phi_2)$
then
$\displaystyle z_1z_2 = \rho_1\rho_2[\cos(\phi_1 + \phi_2) + i\sin(\phi_1 + \phi_2)]\\\vspace{0.2 in} \frac{z_1}{z_2} = \frac{\rho_1}{\rho_2}[\cos(\phi_1 - \phi_2) + i\sin(\phi_1 - \phi_2)]$
Also if n is any real number, you have
$\displaystyle z^n = [\rho(\cos\phi + i\sin\phi)]^n = \rho^n(\cos n\phi + i\sin n\phi)$
which is often called De Moivre’s theorem. You can use this to determine roots of complex numbers. For example if n is a positive integer,
$\displaystyle z^{\frac{1}{n}} = [\rho(\cos\phi + i\sin\phi)]^\frac{1}{n} = \rho^{\frac{1}{n}}\left\{\cos\left(\frac{\phi + 2k\pi}{n}\right) + i\sin\left(\frac{\phi + 2k\pi}{n}\right)\right\}\\\vspace{0.2 in} \ \ k = 0,\ 1,\ 2,\ \cdots,\ n-1$
Using the series for e^x, sin x, cos x, you are led to define
$\displaystyle e^{i\phi} = \cos\phi + i\sin\phi,\ e^{-i\phi} = \cos\phi - i\sin\phi$
which are called Euler’s formulas and which enable you to rewrite equations in terms of exponentials.

複素数

　複素数は $x^2 + 1 = 0$ や $x^2 + x + 1 = 0$ といった実数解を持たない整方程式を解く際に出現します．複素数は a + bi という形をしており，a, b は実数部であり，i は虚数部と呼ばれ，i² = -1 という性質を持ちます．複素数は以下のように定義されます．

加算
$\displaystyle (a + bi) + (c + di) = (a + c) + (b + d)i$
減算
$\displaystyle (a + bi) - (c + di) = (a - c) + (b - d)i$
乗算
$\displaystyle (a + bi)\times(c + di) = ac + adi + bci + bdi^2 = (ac - bd) + (ad + bc)i$
除算
$\displaystyle \frac{a + bi}{c + di} = \frac{a + bi}{c + di}\times\frac{c - di}{c - di} = \frac{ac + bd}{c^2 + d^2} + \frac{bc - ad}{c^2 + d^2}i$

　いかなる状況でも i² を -1 で置換することを除けば，代数の通常の定義が用いられます．交換法則，結合法則，分配法則もまた複素数に適用されます．a + bi の a および b はそれぞれ実数部および虚数部と呼ばれます．二つの複素数が等しいとは，実数部と虚数部とがそれぞれ等しい時に限られます．

　ある複素数 z = x + iy は xy 直交平面上の (x, y) 座標の点 P とみなすことができ，この場合この平面を複素平面またはアルガン図と呼びます．仮に原点 O から点 P への直線を引き，OP 間の距離を ρ とし，OP と x 軸のなす角度を φ とすると，次の図を得ます．

$\displaystyle x = \rho \cos\phi,\ y = \rho\sin\phi,\ \rho = \sqrt{x^2 + y^2}$

また複素数をいわゆる極座標系式として以下のように記述できます．

$\displaystyle z = x + iy = \rho(\cos\phi + i\sin\phi) = \rho cis\phi$

　しばしば ρ は z のモジュラスまたは絶対値と呼ばれ，|z| と記述します．角 φ は z の amplitude または argument と呼ばれ，arg z と略記します． $\rho = \sqrt{z\bar{z}}$ とも記述できます．ただし $\bar{z} = x - iy$ は $z = x + iy$ の共役複素数と呼ばれます．

　極座標で複素数を記述すると以下のようになります．

$\displaystyle z_1 = \rho_1(\cos\phi_1 + i\sin\phi_1),\ z_2 = \rho_2(\cos\phi_2 + i\sin\phi_2)$

すると

$\displaystyle z_1z_2 = \rho_1\rho_2[\cos(\phi_1 + \phi_2) + i\sin(\phi_1 + \phi_2)]\\\vspace{0.2 in} \frac{z_1}{z_2} = \frac{\rho_1}{\rho_2}[\cos(\phi_1 - \phi_2) + i\sin(\phi_1 - \phi_2)]$

　仮に n を任意の実数とすると以下を得ます．

$\displaystyle z^n = [\rho(\cos\phi + i\sin\phi)]^n = \rho^n(\cos n\phi + i\sin n\phi)$

これはド・モアブルの定理として知られています．これを用いて複素数の根を定義できます．例えば仮に n を正の整数と仮定すると，

$\displaystyle z^{\frac{1}{n}} = [\rho(\cos\phi + i\sin\phi)]^\frac{1}{n} = \rho^{\frac{1}{n}}\left\{\cos\left(\frac{\phi + 2k\pi}{n}\right) + i\sin\left(\frac{\phi + 2k\pi}{n}\right)\right\}\\\vspace{0.2 in} \ \ k = 0,\ 1,\ 2,\ \cdots,\ n-1$

e^x, sin x, cos x のための級数を用いると以下の定義に導かれます．

$\displaystyle e^{i\phi} = \cos\phi + i\sin\phi,\ e^{-i\phi} = \cos\phi - i\sin\phi$

これらはオイラーの公式と呼ばれ，方程式を指数関数の観点から書き直すことができます．

Leibnitz’s rule for differentiating an integral

Let
$\displaystyle I(a) = \int_{a}^{b}f(x, \alpha)dx$
where f is supposed continuous and differentiable. Then Leibnitz’s rule states that if a and b are differentiable functions of α,
$\displaystyle \frac{dI}{d\alpha} = \int_{a}^{b}\frac{\partial f}{\partial \alpha}dx + f(b, \alpha)\frac{db}{d\alpha} - f(a, \alpha)\frac{da}{d\alpha}$

積分を微分するためのライプニッツの法則

　今，

$\displaystyle I(a) = \int_{a}^{b}f(x, \alpha)dx$

であるとし，f が連続で微分可能であると仮定します．するとライプニッツの法則により，もし a と b とが共に α の関数を微分可能である時には以下のことが言えます．

$\displaystyle \frac{dI}{d\alpha} = \int_{a}^{b}\frac{\partial f}{\partial \alpha}dx + f(b, \alpha)\frac{db}{d\alpha} - f(a, \alpha)\frac{da}{d\alpha}$

Method of Lagrange multipliers

Sometimes you may wish to find the relative maxima or minima of f(x, y) = 0 subject to some constraint condition φ(x, y) = 0. To do this, form the function h(x, y) = f(x, y) + λφ(x, y) and set
$\displaystyle \partial h/\partial x = 0,\ \partial h/\partial y = 0$
The constant λ is called a Lagrange multiplier and the method is called the method of Lagrange multipliers.

ラグランジュの未定乗数法

　 φ(x, y) = 0 という制約条件のもとで f(x, y) = 0 の極大と極小を求めたくなるかもしれません．このために h(x, y) = f(x, y) + λφ(x, y) という関数を置き，次のようにします．

$\displaystyle \partial h/\partial x = 0,\ \partial h/\partial y = 0$

　定数 λ はラグランジュ未定乗数と呼ばれ，この方法をラグランジュ未定乗数法と呼びます．

Maxima and minima

If for all x such that |x – a| < δ where f(x) ≤ f(a) [ or f(x) ≥ f(a)], f(a) is a relative maximam [ or relative minimum]. For f(x) to have a relative maximum or minimum at x = a, it must have f'(a) = 0. Then if f”(a) < 0 it is a relative maximum while if f”(a) ≥ 0 it is a relative minimum. Possible points at which f(x) has a relative maxima or minima are obtained by solving f'(x) = 0, i.e. by finding the values of x where the slope of the graph f(x) is equal to zero.

Similarly f(x, y) has a relative maximum or minimum at x = a, y = b if f_x(a, b) = 0, f_y(a, b) = 0. Thus possible points at which f(x, y) has relative maxima or minima are obtained by solving simultaneously the equations
$\displaystyle \frac{\partial f}{\partial x} = 0,\ \frac{\partial f}{\partial y} = 0$
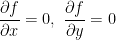
Extensions to functions of more than two variables are similar.

極大と極小

　全ての x について |x – a| < δ であり，また f(x) ≤ f(a) （または f(x) ≥ f(a)）である時，f(a) は極大（または極小）であると言います．

　f(x) が x = a において極大または極小を持つには f'(a) = 0 でなくてはなりません．もし f”(a) < 0 ならそれは極大であり，一方もし f”(a) > 0 ならそれは極小です．f(x) において極大または極小となる可能性のある点は f'(x) = 0 を解くこと，例えば， f(x) のグラフの傾きがゼロと等しくなる x の値を見つけることで得られます．

　同様に f_x(a, b) = 0, f_y(a, b) = 0 ならば f(x, y) は x = a, y = b において極大または極小を持ちます．故に f(x, y) f(x, y) で極大または極小をもつ可能性のある点は，同様に次の方程式を解くことで得られます．

$\displaystyle \frac{\partial f}{\partial x} = 0,\ \frac{\partial f}{\partial y} = 0$

　2 変数以上の関数への拡張も同様です．

Linear equations and determinants

$\displaystyle a_1x + b_1y = c_1\\\vspace{0.2 in} a_2x + b_2y = c_2\ \cdots(1)$
These represent two lines in the xy plane, and in general will meet in a point whose coordinates (x, y) are found by solving simultaneously.
$\displaystyle x = \frac{c_1b_2 - b_1c_2}{a_1b_2 - b_1a_2},\ y = \frac{a_1c_2 - c_1a_2}{a_1b_2 - b_1a_2}\ \cdots(2)$
It’s convenient to write these in determinant form as
$\displaystyle x = \frac{\left|\begin{array}{cc}c_1 & b_1 \\ c_2 & b_2\end{array}\right|}{\left|\begin{array}{cc}a_1 & b_1 \\ a_2 & b_2\end{array}\right|},\ y = \frac{\left|\begin{array}{cc}a_1 & c_1 \\ a_2 & c_2\end{array}\right|}{\left|\begin{array}{cc}a_1 & b_1 \\ a_2 & b_2 \end{array}\right|}\ \cdots(3)$
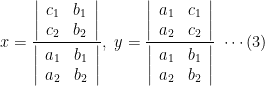
where it is defined a determinant of the second order or order 2 to be
$\displaystyle \left|\begin{array}{cc}a & b \\ c & d \end{array}\right| = ad - bc\ \cdots(4)$
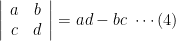
It should be noted that the denominator for x and y in (3) is the determinant consisting of the coefficients of x and y in (1). The numerator for x is found by replacing the first column of the denominator by the constants c₁, c₂ on the right side of (1). Similarly the numerator for y is found by replacing the second column of the denominator by c₁, c₂. This procedure is often called Cramer’s rule. In case the denominator in (3) is zero, the two lines represented by (1) do not meet in one point but are either coincident or parallel.

The ideas are easily extended. Thus you can consider the equations
$\displaystyle a_1x + b_1y + c_1z = d_1\\\vspace{0.2 in} a_2x + b_2y + c_2z = d_2\ \cdots(5)\\\vspace{0.2 in} a_3x + b_3y + c_3z = d_3$
representing 3 planes. If they intersect in a point, the coordinates (x, y, x) of this point are found from Cramer’s rule to be
$\displaystyle x = \frac{\left|\begin{array}{ccc}d_1 & b_1 & c_1 \\ d_2 & b_2 & c_2 \\ d_3 & b_3 & c_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|},\ y = \frac{\left|\begin{array}{ccc}a_1 & d_1 & c_1 \\ a_2 & d_2 & c_2 \\ a_3 & d_3 & c_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|},\ z = \frac{\left|\begin{array}{ccc}a_1 & b_1 & d_1 \\ a_2 & b_2 & d_2 \\ a_3 & b_3 & d_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|}\ \cdots(6)$
where it can be defined the determinant of order 3 by
$\displaystyle \left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\a_3 & b_3 & c_3\end{array}\right| = a_1b_2c_3 + b_1c_2a_3 + c_1a_2b_3 - (b_1a_2c_3 + a_1c_2b_3 + c_1b_2a_3)\ \cdots(7)$
The determinant can also be evaluated in terms of second order determinants as follows
$\displaystyle a_1\left|\begin{array}{cc}b_2 & c_2 \\ b_3 & c_3\end{array}\right| - b_1\left|\begin{array}{cc}a_2 & c_2 \\ a_3 & c_3\end{array}\right| + c_1\left|\begin{array}{cc}a_2 & b_2 \\ a_3 & b_3\end{array}\right|\ \cdots(8)$
where it is noted that a₁, b₁, c₁ are the elements in the first row and the corresponding second order determinants are those obtained from the given third order determinant by removing the row and column in which the element appears.

一次方程式と行列式

$\displaystyle a_1x + b_1y = c_1\\\vspace{0.2 in} a_2x + b_2y = c_2\ \cdots(1)$

　これらは xy 平面における 2 本の直線を示しており，一般に (x, y) 座標で交わる 1 点において同時に解が得られます．

$\displaystyle x = \frac{c_1b_2 - b_1c_2}{a_1b_2 - b_1a_2},\ y = \frac{a_1c_2 - c_1a_2}{a_1b_2 - b_1a_2}\ \cdots(2)$

　これを行列式で表現するのは便利です．

$\displaystyle x = \frac{\left|\begin{array}{cc}c_1 & b_1 \\ c_2 & b_2\end{array}\right|}{\left|\begin{array}{cc}a_1 & b_1 \\ a_2 & b_2\end{array}\right|},\ y = \frac{\left|\begin{array}{cc}a_1 & c_1 \\ a_2 & c_2\end{array}\right|}{\left|\begin{array}{cc}a_1 & b_1 \\ a_2 & b_2 \end{array}\right|}\ \cdots(3)$

　2 次の行列式は次のように定義します．

$\displaystyle \left|\begin{array}{cc}a & b \\ c & d \end{array}\right| = ad - bc\ \cdots(4)$

　強調すべきことですが，(3) で記述した x と y の分母は (1) の x と y の係数を含む行列式です．x の分子は分母の 1 列目を (1) の右側の c₁, c₂ の定数で置換して得られます．同様に y の分子は c₁, c₂ で 2 列目を置換して得られます．この処理はしばしば Crame’s rule と呼ばれます．(3) の分母がゼロの場合は (1) で示される 2 行は1点で交差せず，一致するか平行であるかです．

　この考えは容易に拡張できます．次の方程式を考えてみましょう．

$\displaystyle a_1x + b_1y + c_1z = d_1\\\vspace{0.2 in} a_2x + b_2y + c_2z = d_2\ \cdots(5)\\\vspace{0.2 in} a_3x + b_3y + c_3z = d_3$

3行を示します．これらが 1 点で交わる場合，この点の (x, y, z) 座標は Cramer’s rule から得られます．

$\displaystyle x = \frac{\left|\begin{array}{ccc}d_1 & b_1 & c_1 \\ d_2 & b_2 & c_2 \\ d_3 & b_3 & c_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|},\ y = \frac{\left|\begin{array}{ccc}a_1 & d_1 & c_1 \\ a_2 & d_2 & c_2 \\ a_3 & d_3 & c_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|},\ z = \frac{\left|\begin{array}{ccc}a_1 & b_1 & d_1 \\ a_2 & b_2 & d_2 \\ a_3 & b_3 & d_3\end{array}\right|}{\left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\ a_3 & b_3 & c_3\end{array}\right|}\ \cdots(6)$

　3 次の行列式は次のように定義されます．

$\displaystyle \left|\begin{array}{ccc}a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2 \\a_3 & b_3 & c_3\end{array}\right| = a_1b_2c_3 + b_1c_2a_3 + c_1a_2b_3 - (b_1a_2c_3 + a_1c_2b_3 + c_1b_2a_3)\ \cdots(7)$

　この行列式は 2 次の行列式の面で次のように評価されます．

$\displaystyle a_1\left|\begin{array}{cc}b_2 & c_2 \\ b_3 & c_3\end{array}\right| - b_1\left|\begin{array}{cc}a_2 & c_2 \\ a_3 & c_3\end{array}\right| + c_1\left|\begin{array}{cc}a_2 & b_2 \\ a_3 & b_3\end{array}\right|\ \cdots(8)$

　ここで強調しておきたいことは，a₁, b₁, c₁ は 1 行目の要素であり，対応する 2 次の行列式は 3 次の行列式からその要素が現れる行と列を除去して得られます．

Taylor series for functions of two or more variables

The ideas involved in Taylor series for functions of one variable can be generalized. For example, the Taylor series for $f(x, y)$ about x = a, y = b can be written
$\displaystyle f(x, y) = f(a, b) + f_x(a, b)(x -a) + f_y(a, b)(y -b)\\\vspace{0.2 in} \ \ \ + \frac{1}{2!}\big[f_{xx}(a, b)(x - a)^2 + 2f_{xy}(a, b)(x - a)(y - b) + f_{yy}(a, b)(y - b)^2\big] + \cdots$

2変数以上の関数のテーラー級数

　1 変数関数のテーラー級数に関する考えは一般化可能です．例えば， $f(x, y$ の x = a, y = b におけるテーラー級数は下記のように記述できます．

$\displaystyle f(x, y) = f(a, b) + f_x(a, b)(x -a) + f_y(a, b)(y -b)\\\vspace{0.2 in} \ \ \ + \frac{1}{2!}\big[f_{xx}(a, b)(x - a)^2 + 2f_{xy}(a, b)(x - a)(y - b) + f_{yy}(a, b)(y - b)^2\big] + \cdots$

Partial derivatives

The partial derivatives of $f(x, y)$ with respect to x and y are defined by
$\displaystyle \frac{\partial f}{\partial x}= \lim\limits_{h \rightarrow 0} \frac{f(x + h, y) - f(x, y)}{h}\\\vspace{0.2 in} \frac{\partial f}{\partial y} = \lim\limits_{k \rightarrow 0} \frac{f(x, y + k) - f(x, y)}{k}$
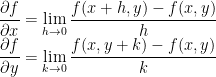
if these limits exist. It’s often written h = Δx, k = Δy. Note that $\partial f/\partial x$ is simply the ordinary derivative of f with respect to x keeping y constant, while $\partial f/\partial y$ is the ordinary derivative of f with respect to y keeping x constant.

Higher derivatives are defined similarly. For example, you have the second order derivatives
$\displaystyle \frac{\partial}{\partial x}\left(\frac{\partial f}{\partial x}\right) = \frac{\partial^2f}{\partial x^2}\\\vspace{0.2 in} \frac{\partial}{\partial x}\left(\frac{\partial f}{\partial y}\right) = \frac{\partial^2f}{\partial x\partial y}\\\vspace{0.2 in} \frac{\partial}{\partial y}\left(\frac{\partial f}{\partial x}\right) = \frac{\partial^2f}{\partial y\partial x}\\\vspace{0.2 in} \frac{\partial}{\partial y}\left(\frac{\partial f}{\partial y}\right) = \frac{\partial^2f}{\partial y^2}$
The deviation are sometimes denoted f_x and f_y. In such case f_x(a, b), f_y(a, b) denote these partial derivatives evaluated at (a, b).

The deviations are denoted by f_xx, f_xy, f_yx, f_yy respectively. The second and third results will be the same if f has continuous partial derivatives of second order at least.

The differentiation of f(x, y) is defined as
$\displaystyle df = \frac{\partial f}{\partial x}dx + \frac{\partial f}{\partial y}dy$
where h = Δx = dx, k = Δy = dy.

偏微分

　x および y に対しての $f(x, y)$ の偏導関数は次式で定義されます．

$\displaystyle \frac{\partial f}{\partial x}= \lim\limits_{h \rightarrow 0} \frac{f(x + h, y) - f(x, y)}{h}\\\vspace{0.2 in} \frac{\partial f}{\partial y} = \lim\limits_{k \rightarrow 0} \frac{f(x, y + k) - f(x, y)}{k}$

　しばしば h = Δx, k = Δy のように記述します．y を定数とした x に対する f の通常の導関数は単に $\partial f/\partial x$ と記述し，一方 x を定数とした y に対する f の通常の導関数は $\partial f/\partial y$ と記述します．

　高階の導関数もまた同様に定義します．例えば，2 階の通常の導関数は下記のようです．

$\displaystyle \frac{\partial}{\partial x}\left(\frac{\partial f}{\partial x}\right) = \frac{\partial^2f}{\partial x^2}\\\vspace{0.2 in} \frac{\partial}{\partial x}\left(\frac{\partial f}{\partial y}\right) = \frac{\partial^2f}{\partial x\partial y}\\\vspace{0.2 in} \frac{\partial}{\partial y}\left(\frac{\partial f}{\partial x}\right) = \frac{\partial^2f}{\partial y\partial x}\\\vspace{0.2 in} \frac{\partial}{\partial y}\left(\frac{\partial f}{\partial y}\right) = \frac{\partial^2f}{\partial y^2}$

　偏導関数は時々 f_x や f_y とも記述します．そのような場合 f_x(a, b), f_y(a, b) は点 (a, b) において評価されるこれらの偏微分です．

　偏導関数はまた f_xx, f_xy, f_yx, f_yy とも記述します．f が少なくとも 2 階の連続な偏微分を有するなら 2 階や 3 階微分の結果もまた同様です．

　 $f(x, y)$ の全微分は次のように定義します．

$\displaystyle df = \frac{\partial f}{\partial x}dx + \frac{\partial f}{\partial y}dy$

　ただし h = Δx = dx, k = Δy = dy です．

Functions of two or more variables

The concept of function of one variable can be extended to functions of two or more variables. Thus for example $z = f(x, y)$ defines a function f which assigns to the number pair (x, y) the number z.

It’s familiar for some people with graphing $z = f(x, y)$ in a 3-dimensional xyz coordinate system to obtain a surface. Sometime x and y are called independent variables and z a dependent variable. Occasionally it’s written $z = z(x, y)$ rather than $z = f(x, y)$ , using the system z in two different senses. however, no confusion should result.

The ideas of limits and continuity for functions of two or more variables pattern closely those for one variable.

2変数以上の関数

　1 変数関数の概念は 2 変数以上の関数にも拡張可能です．それゆえ例えば $z = f(x, y)$ は 2 つの数 (x, y) を 1 つの数 z に割り付ける関数 f を定義するものです．

　ある人にとっては $z = f(x, y)$ を xyz 座標軸系の 3 次元にグラフ化して面を得ることは馴染み深いでしょう．時には x と y は独立変数と呼ばれ，z は従属変数と呼ばれます．まれに $z = f(x, y)$ ではなく $z = z(x, y)$ と記述されることがあり，z 系は異なる意味で用いられます．しかし混同すべきではありません．

　2 変数以上の関数の極限と連続性の概念は 1 変数のそれに近いです．

Taylor series

The Taylor series for f(x) about x = a is defined as

$\displaystyle f(x) = f(a) + f'(a)(x - a) + \frac{f''(a)(x - a)^2}{2!} + \cdots + \frac{f^{n -1}(a)(x - a)^{n-1}}{(n -1)!} + R_n$ (a)
where $\displaystyle R_n = \frac{f^n(x - n)^n}{n!}$ , x₀ between a and x.(b)
is called the reminder and where it is supposed that f(x) has derivatives of order n at least. The case where n = 1 is often called law of the mean or mean-value theorem and can be written as
$\displaystyle \frac{f(x) -f(a)}{x - a} = f'(x_0)$ , x₀ between a and x (c)

The infinite series corresponding to (a), also called the formal Taylor series for f(x), will converge in some interval if $\lim\limits_{n \rightarrow \infty}R_n = 0$ in this interval. Some important Taylor series together with their intervals of convergence are as follows.

$\displaystyle e^n = 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} +\frac{x^4}{4!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \sin x = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \cos x = 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \ln(1 + x) = x - \frac{x^2}{2!} + \frac{x^3}{3!} - \frac{x^4}{4!} + \cdots\ -1 < x \le 1[/latex]</li> <li>[latex]\displaystyle \tan^{-1}x = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots\ -1 \le x \le 1$

A series of the form $\sum_{n=0}^{\infty}c_n(x - a)^n$ is often called a power series. Such power series are uniformly convergent in any interval which lies entirely within the interval of convergence.

テーラー級数

　x = a における f(x) のテーラー級数は下記のように定義されます．

$\displaystyle f(x) = f(a) + f'(a)(x - a) + \frac{f''(a)(x - a)^2}{2!} + \cdots + \frac{f^{n -1}(a)(x - a)^{n-1}}{(n -1)!} + R_n$ (a)
ここで $\displaystyle R_n = \frac{f^n(x - n)^n}{n!}$ , x₀ は a と x の範囲内(b)
は reminder と呼ばれ， f(x) が最低でも n 次の微分係数を持つと想定します．n = 1 の場合は特に平均の法則または平均値の定理と呼ばれ，次のように記述します．
$\displaystyle \frac{f(x) -f(a)}{x - a} = f'(x_0)$ , x₀ は a と x の範囲内(c)

　(a) に対応した無限級数は f(x) の正規テーラー級数とも呼ばれ，必ずある区間に収束します．仮に $\lim\limits_{n \rightarrow \infty}R_n = 0$ がこの区間にあるなら．いくつかの重要なテーラー級数と収束値をその区間とともに示します．

$\displaystyle e^n = 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} +\frac{x^4}{4!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \sin x = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \cos x = 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \frac{x^6}{6!} + \cdots\ -\infty < x < \infty[/latex]</li> <li>[latex]\displaystyle \ln(1 + x) = x - \frac{x^2}{2!} + \frac{x^3}{3!} - \frac{x^4}{4!} + \cdots\ -1 < x \le 1[/latex]</li> <li>[latex]\displaystyle \tan^{-1}x = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots\ -1 \le x \le 1$

　ある形の級数 $\sum_{n=0}^{\infty}c_n(x - a)^n$ はしばしばべき級数と呼ばれます．そのようなべき級数はどの区間にも一様収束し，それは収束区間内全体にあります．

月	火	水	木	金	土	日
						1
2	3	4	5	6	7	8
9	10	11	12	13	14	15
16	17	18	19	20	21	22
23	24	25	26	27	28	29
30	31