2013/12/12 | Improve Society

Polynomials

Polynomial is formula as below;
$\displaystyle f(x) = a_0x^n + a_1x^{n-1} + a_2x^{n-2} + \cdots + a_n$
If $a_0 \neq 0$ , n is called as degree of polynomials.
$\displaystyle (a + x)^n = a^n + \left(\frac{n}{1}\right)a^{n -1}x + \left(\frac{n}{2}\right)a^{n -2}x^2 + \cdots + x^n$
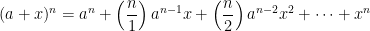
where the binomial coefficients are given by

$\displaystyle \left(\frac{n}{k}\right) = \frac{n!}{k!(n - k)!}$
and where factorial n, i.e. n! = n(n -1)(n-2)…1 while 0! = 1 by definition.

Exponential function
$\displaystyle f(x) = a^x$
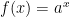
An important special case occurs where a = e = 2.718…

Exponential law

$\displaystyle a^{m + n} = a^m \cdot a^n$

$\displaystyle a^{m - n} = \frac{a^m}{a^n},\ a \neq 0$

$\displaystyle (a^m)^n = a^{mn}$

Logarithmic function
$\displaystyle f(x) = \log_a x$
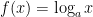
These functions are inverse of the exponential functions, i.e. if a^x = y then x = log_ay where a is called the base of the logarithm. If a = e, which is often called the natural base of logarithm, it’s described log_e by ln x, called the natural logarithm of x.

Logarithmic law

$\displaystyle \ln(mn) = \ln(m) + \ln(n)$

$\displaystyle \ln\frac{m}{n} = \ln(m) - \ln(n)$

$\displaystyle \ln{m^p} = p\ln{m}$