日: 2013年12月23日

If for all x such that |x – a| < δ where f(x) ≤ f(a) [ or f(x) ≥ f(a)], f(a) is a relative maximam [ or relative minimum]. For f(x) to have a relative maximum or minimum at x = a, it must have f'(a) = 0. Then if f”(a) < 0 it is a relative maximum while if f”(a) ≥ 0 it is a relative minimum. Possible points at which f(x) has a relative maxima or minima are obtained by solving f'(x) = 0, i.e. by finding the values of x where the slope of the graph f(x) is equal to zero.

Similarly f(x, y) has a relative maximum or minimum at x = a, y = b if f_x(a, b) = 0, f_y(a, b) = 0. Thus possible points at which f(x, y) has relative maxima or minima are obtained by solving simultaneously the equations

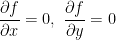
Extensions to functions of more than two variables are similar.

　全ての x について |x – a| < δ であり，また f(x) ≤ f(a) （または f(x) ≥ f(a)）である時，f(a) は極大（または極小）であると言います．

　f(x) が x = a において極大または極小を持つには f'(a) = 0 でなくてはなりません．もし f”(a) < 0 ならそれは極大であり，一方もし f”(a) > 0 ならそれは極小です．f(x) において極大または極小となる可能性のある点は f'(x) = 0 を解くこと，例えば， f(x) のグラフの 傾き がゼロと等しくなる x の値を見つけることで得られます．

　同様に f_x(a, b) = 0, f_y(a, b) = 0 ならば f(x, y) は x = a, y = b において極大または極小を持ちます．故に f(x, y) f(x, y) で極大または極小をもつ可能性のある点は，同様に次の方程式を解くことで得られます．

　2 変数以上の関数への拡張も同様です．