日: 2014年1月4日

Special first order equations and solutions

Any first order differential equation can be put into the form

\displaystyle \frac{dy}{dx} = f(x,y)

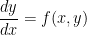
or

\displaystyle M(x,y)dx + N(x,y)dy = 0

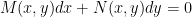
and the general solution of such an equation contains one arbitrary constant. Many special devices are available for finding general solutions of various types of first order differential equations. In the following list some of types are given.

  1. Separation of variables
  2. Exact equation
  3. Integrating factor
  4. Linear equation
  5. Homogeneous equation
  6. Bernoulli’s equation
  7. Equation solvable for y
  8. Clairaut’s equation
  9. Miscellaneous equations

1. Separation of variables

If differential equation is given as below,

\displaystyle f_1(x)g_1(y)dx + f_2(x)g_2(y)dy = 0

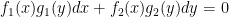
divide by g_1(y)f_2(x) \ne 0 and integrate to obtain general solution

\displaystyle \int\frac{f_1(x)}{f_2(x)}dx + \int\frac{g_2(y)}{g_1(y)}dy = c

2. Exact equation

If differential equation is given as below,

\displaystyle M(x, y)dx + N(x, y)dy = 0

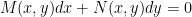
where \displaystyle \frac{\partial M}{\partial y} = \frac{\partial N}{\partial x}

The equation can be written as

\displaystyle Mdx + Ndy = dU(x, y) = 0

where dU is an exact differential. Thus the solution is U(x, y) = c or equivalently

\displaystyle \int M\partial x + \int\left(N - \frac{\partial}{\partial y}\int M\partial x\right)dy = c

where δx indicates that the integration is to be performed with respect to x keeping y constant.

3. Integrating factor

If differential equation is given as below,

\displaystyle M(x, y)dx + N(x, y)dy = 0

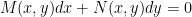
where

\displaystyle \frac{\partial M}{\partial y} \neq \frac{\partial N}{\partial x}

The equation can be written as an exact differential equation

\displaystyle \mu M dx + \mu N dy = 0

where μ is an appropriate integrating factor.

The following combination are often useful in finding integration factors.

\displaystyle \frac{xdy - ydx}{x^2} = d\left(\frac{y}{x}\right)
\displaystyle \frac{xdy - ydx}{y^2} = -d\left(\frac{x}{y}\right)
\displaystyle \frac{xdy - ydx}{x^2 + y^2} = d\left(\tan^{-1}\frac{y}{x}\right)
\displaystyle \frac{xdy - ydx}{x^2 - y^2} = \frac{1}{2}d\left(\ln\frac{x - y}{x + y}\right)
\displaystyle \frac{xdx + ydy}{x^2 + y^2} = \frac{1}{2}d\{\ln(x^2 + y^2)\}

4. Linear equation

If differential equation is given as below,

\displaystyle \frac{dy}{dx} + P(x)y = Q(x)

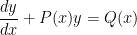
An integrating factor is given by

\displaystyle \mu = e^{\int P(x)dx}

and the equation can then be written

\displaystyle \frac{d}{dx}(\mu y) = \mu Q

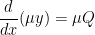
with solution

\displaystyle \mu y = \int \mu Qdx + c

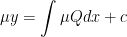
or

\displaystyle ye^{\int Pdx} = \int Qe^{\int Pdx}dx + c

5. Homogeneous equation

If differential equation is given as below,

\displaystyle \frac{dy}{dx} = F\left(\frac{y}{x}\right)

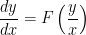
Let y/x = v or y = vx, and the equation becomes

\displaystyle v + x\frac{dv}{dx} + F(x)

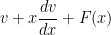
or

\displaystyle xdv + (F(x) - v)dx = 0

which is of Type 1 and has the solution

\displaystyle \ln x = \int \frac{dv}{F(v) - v} + c

where v = y/x. If F(v) = v, the solution is y = cx.

6. Bernoulli’s equation

If differential equation is given as below,

\displaystyle \frac{dy}{dx} + P(x)y = Q(x)y^n,\ n \neq 0, 1

Letting v = y^{1 - n}, the equation reduces to Type 4 with solution

\displaystyle ve^{(1-n)\int Pdx} = (1 - n)\int Qe^{(1-n)\int Pdx}dx + c

If n = 0, the equation is of Type 4. If n = 1, it is of Type 1.

7. Equation solvable for y

If differential equation is given as below,

\displaystyle y = g(x, y)

where

\displaystyle p = y'

Differentiate both sides of the equation with respect to x to obtain

\displaystyle \frac{dy}{dx} = \frac{dg}{dx} = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}

or

\displaystyle p = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}

Then solve this last equation to obtain G(x, p, c) = 0. The required solution is obtained by eliminating p between G(x, p, c) = 0 and y = g(x, p).

An analogous method exists if the equation is solvable for x.

8. Clairaut’s equation

If differential equation is given as below,

\displaystyle y = px + F(p)

where

\displaystyle p = y'

The equation is of Type 7 and has solution

\displaystyle y = cx + F(c)

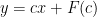
The equation will also have a singular solution in general.

9. Miscellaneous equations

If differential equation is given as below,

\displaystyle (a) \frac{dy}{dx} = F(\alpha x + \beta y)\\\vspace{0.2 in} (b) \frac{dy}{dx} = F\left(\frac{\alpha_1 x + \beta_1 y + \gamma_1}{\alpha_2 x + \beta_2 y + \gamma_2}\right)

(a)Letting \alpha x + \beta y = v, the equation reduces Type 1.

(b)Let x = X +h,\ y = Y + k and choose constants h and k so that the equation reduces to Type 5. This is possible if and only if \alpha_1/\alpha_2 \neq \beta_1/\beta_2. If \alpha_1/\alpha_2 = \beta_1/\beta_2, the equation reduces to Type 9(a).

特殊な1階常微分方程式とその解

　いかなる 1 階の微分方程式も次の形に置き換えることができます．

\displaystyle \frac{dy}{dx} = f(x,y)

または

\displaystyle M(x,y)dx + N(x,y)dy = 0

そしてそれらの方程式の一般解は一つの任意定数を持ちます．様々な種類の 1 階微分方程式の一般解の発見には多くの特殊な装置が有用です．下記のリストにはその幾つかを示してあります．

  1. 変数分離法
  2. 完全微分方程式
  3. 積分因子
  4. 線形微分方程式
  5. 同次方程式
  6. ベルヌーイの方程式
  7. y について解ける方程式
  8. クレローの方程式
  9. その他の方程式

1. 変数分離法

　微分方程式が下記のようである場合は

\displaystyle f_1(x)g_1(y)dx + f_2(x)g_2(y)dy = 0

一般解を得るには g_1(y)f_2(x) \ne 0 で除し，積分します．

\displaystyle \int\frac{f_1(x)}{f_2(x)}dx + \int\frac{g_2(y)}{g_1(y)}dy = c

2. 完全微分方程式

　微分方程式が下記のようである場合は

\displaystyle M(x, y)dx + N(x, y)dy = 0

ここで \displaystyle \frac{\partial M}{\partial y} = \frac{\partial N}{\partial x}

　この方程式は次のように書き換えられます．

\displaystyle Mdx + Ndy = dU(x, y) = 0

ここで dU は完全微分方程式です．ゆえにその解は U(x, y) = c または同等の解として

\displaystyle \int M\partial x + \int\left(N - \frac{\partial}{\partial y}\int M\partial x\right)dy = c

ここで δx は y を定数とし x による積分を行うことを示します．

3. 積分因子

　微分方程式が下記のようである場合，

\displaystyle M(x, y)dx + N(x, y)dy = 0

ここで

\displaystyle \frac{\partial M}{\partial y} \neq \frac{\partial N}{\partial x}

　この方程式は次のように完全微分方程式に書き換えられます．

\displaystyle \mu M dx + \mu N dy = 0

ここで μ は適切な積分因子です．

　下記の組み合わせは積分因子を発見するのにしばしば有用です．

\displaystyle \frac{xdy - ydx}{x^2} = d\left(\frac{y}{x}\right)
\displaystyle \frac{xdy - ydx}{y^2} = -d\left(\frac{x}{y}\right)
\displaystyle \frac{xdy - ydx}{x^2 + y^2} = d\left(\tan^{-1}\frac{y}{x}\right)
\displaystyle \frac{xdy - ydx}{x^2 - y^2} = \frac{1}{2}d\left(\ln\frac{x - y}{x + y}\right)
\displaystyle \frac{xdx + ydy}{x^2 + y^2} = \frac{1}{2}d\{\ln(x^2 + y^2)\}

4. 線形微分方程式

　微分方程式が下記のようである場合，

\displaystyle \frac{dy}{dx} + P(x)y = Q(x)

　積分因子は次のように得られます．

\displaystyle \mu = e^{\int P(x)dx}

また方程式は次のように書き換えられます．

\displaystyle \frac{d}{dx}(\mu y) = \mu Q

解は以下のようです．

\displaystyle \mu y = \int \mu Qdx + c

または

\displaystyle ye^{\int Pdx} = \int Qe^{\int Pdx}dx + c

5. 同次方程式

微分方程式が下記のようである場合，

\displaystyle \frac{dy}{dx} = F\left(\frac{y}{x}\right)

　y/x = v または y = vx とします．すると方程式は次のようになります．

\displaystyle v + x\frac{dv}{dx} + F(x)

または

\displaystyle xdv + (F(x) - v)dx = 0

ここで Type 1 と同じになり，解は次のようになります．

\displaystyle \ln x = \int \frac{dv}{F(v) - v} + c

ここで v = y/x です．もし F(v) = v なら解は y = cx となります．

6. ベルヌーイの方程式

微分方程式が下記のようである場合，

\displaystyle \frac{dy}{dx} + P(x)y = Q(x)y^n,\ n \neq 0, 1

　v = y^{1 - n} とします．すると方程式は Type 4 に置き換えられ，解は次のようになります．

\displaystyle ve^{(1-n)\int Pdx} = (1 - n)\int Qe^{(1-n)\int Pdx}dx + c

　仮に n = 0 なら方程式は Type 4 と同じであり， n = 1 なら Type 1 と同じです．

7. y について解ける方程式

　微分方程式が下記のようである場合，

\displaystyle y = g(x, y)

ここで

\displaystyle p = y'

　方程式の両辺を x について微分すると次が得られます．

\displaystyle \frac{dy}{dx} = \frac{dg}{dx} = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}

または

\displaystyle p = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}

　そしてこの最後の方程式を解くと G(x, p, c) = 0 が得られます．必要な解は G(x, p, c) = 0 と y = g(x, p) の間の p を消去して得られます．

　その方程式を x について解くための類似の方法が存在します．

8. クレローの方程式

　微分方程式が下記のようである場合，

\displaystyle y = px + F(p)

ここで

\displaystyle p = y'

　この方程式は Type 7 に置き換えられ，解は次の通りです．

\displaystyle y = cx + F(c)

　この方程式もまた一般解のうち単解を有します．

9. その他の方程式

微分方程式が下記のようである場合，

\displaystyle (a) \frac{dy}{dx} = F(\alpha x + \beta y)\\\vspace{0.2 in} (b) \frac{dy}{dx} = F\left(\frac{\alpha_1 x + \beta_1 y + \gamma_1}{\alpha_2 x + \beta_2 y + \gamma_2}\right)

(a)\alpha x + \beta y = v とすると，方程式は Type 1 に置き換えられます．

(b)x = X +h,\ y = Y + k とし，定数 h と k を方程式が Type 5 に置き換えられるように定めます．この処理は以下の場合，すなわち \alpha_1/\alpha_2 \neq \beta_1/\beta_2 の場合に限り可能です．仮に \alpha_1/\alpha_2 = \beta_1/\beta_2 の場合，方程式は Type 9(a) に置き換えられます．