日: 2014年4月19日

Determinants

If the matrix A in (1) is a square matrix, then we associate with A a number denoted by

\displaystyle \Delta = \left| \begin{array}{cccc} a_{11} & a_{12} & \cdots & a_{an} \\ a_{21} & a_{22} & \cdots & a_{2n} \\ \cdots & \cdots & \cdots & \cdots \\ a_{n1} & a_{n2} & \cdots & a_{nn} \end{array} \right|\cdots (9)

called the determinant of A of order n, written det(A). In order to define the value of a determinant, we introduce the following concepts.

1. Minor

Given any element a_{jk} of \Delta we associate a new determinant of order (n – 1) obtained by removing all elements of the jth row and kth column called the minor of a_{jk}.

2. Cofactor

If we multiply the minor of a_{jk} by (-1)^{j+k}, the result of the elements in any row [or column] by their corresponding cofactors and is called the Laplace expansion. In symbols,

\displaystyle \det{A} = \sum^{n}_{k=1}a_{jk}A_{jk} \cdots (10)

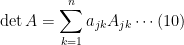
We can show that this value is independent of the row [or column] used.

行列式

　仮に (1) における行列 A が正方行列なら， A に対して下記に示すある数を関連付けられます．

\displaystyle \Delta = \left| \begin{array}{cccc} a_{11} & a_{12} & \cdots & a_{an} \\ a_{21} & a_{22} & \cdots & a_{2n} \\ \cdots & \cdots & \cdots & \cdots \\ a_{n1} & a_{n2} & \cdots & a_{nn} \end{array} \right|\cdots (9)

これは n次 の A の 行列式 と呼び， det(A) と記述します．行列式の値を定義するために次の概念を導入しましょう．

1. 小行列式

\Delta の任意の要素 a_{jk} がある時， j 番目の行および k 番目の列の全要素を除去して得られた (n – 1) 次の新しい行列式を関連付け，これを a_{jk} の小行列式と呼びます．

2. 余因子

　仮に a_{jk} の小行列式に (-1)^{j+k} を乗算するなら，それらの対応する余因子による任意の行（または列）における要素の結果は ラプラス展開 と呼びます．記法では

\displaystyle \det{A} = \sum^{n}_{k=1}a_{jk}A_{jk} \cdots (10)

　この値は用いられる行または列によらず独立です．