日: 2014年8月18日

VECTOR NOTATION FOR LINE INTEGRALS

It is often convenient to express a line integral in vector form as an aid in physical or geometric understanding as well as for brevity of notation. For example, we can express the line integral (15) in the form

\displaystyle \int_{C}[A_1dx + A_2dy + A_3dz] \\ = \int_{C} (A_1\bold{i} + A_2\bold{j} + A_3\bold{k}) \cdot (dx\bold{i} + dy\bold{j} + dz\bold{k})\\ = \int_{C} \bold{A}\cdot d\bold{r} \cdots (17)

where \bold{A} = A_1\bold{i} + A_2\bold{j} + A_3\bold{k} and d\bold{r} = dx\bold{i} + dy\bold{j} + dz\bold{k}. The line integral (14) is a special case of this with z = 0.

If at each point (x, y, z) we associate a force F acting on an object (i.e. if a force field is defined), then

\displaystyle \int_{C} \bold{F}\cdot d\bold{r}\cdots(18)

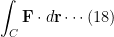
represents physically the total work done in moving the object along the curve C.

線積分のベクトル表記

　簡潔な記法同様，物理学的・地理的な理解のために線積分をベクトルの形で表現することはしばしば便利です．例えば，線積分 (15) を次の形で表現できます．

\displaystyle \int_{C}[A_1dx + A_2dy + A_3dz] \\ = \int_{C} (A_1\bold{i} + A_2\bold{j} + A_3\bold{k}) \cdot (dx\bold{i} + dy\bold{j} + dz\bold{k})\\ = \int_{C} \bold{A}\cdot d\bold{r} \cdots (17)

ここで \bold{A} = A_1\bold{i} + A_2\bold{j} + A_3\bold{k} および d\bold{r} = dx\bold{i} + dy\bold{j} + dz\bold{k} です．線積分 (14) は z = 0 の時の特殊型です．

　各点 (x, y, z) をある物体に作用する力 F と関連付けるなら（例　仮にある 力場 が定義されると)，

\displaystyle \int_{C} \bold{F}\cdot d\bold{r}\cdots(18)

上記は曲線 C に沿ってなされた物理的な総仕事量を表現します．