日: 2014年9月22日

CONDITIONS FOR A LINE INTEGRAL TO BE INDEPENDENT OF THE PATH

  • Theorem 6-1.

    • A necessary and sufficient condition for \displaystyle \int_C [Pdx + Qdy] to be independent of the path C joining any two given points in a region \cal R is that in \cal R

    \partial P/\partial y = \partial Q/\partial x\cdots (23)

    where it is supposed that these partial derivatives are continuous in \cal R.

The condition (23) is also the condition that Pdx + Qdy is an exact differential, i.e. that there exists a function \phi(x, y) such that Pdx + Qdy = d\phi. In such case if the end points of curve C are (x_1, y_1) and (x_2, y_2), the value of the line integral is given by

\displaystyle \int_{(x_1, y_1)}^{(x_2, y_2)}[Pdx + Qdy] = \int_{(x_1, y_1)}^{(x_2, y_2)} d\phi = \phi(x_2, y_2) - \phi(x_1, y_1) \cdots(24)

In particular if (23) holds and C is closed, we have x_1 = x_2,\ y_1 = y_2 and

\displaystyle \oint_C [Pdx + Qdy] = 0\cdots(25)

The results in Theorem 6-1 can be extended to line integrals in space. Thus we have

  • Theorem 6-2.

    • A necessary and sufficient condition for \displaystyle \int_C [A_1dx + A_2dy + A_3dz] to be independent of the path C joining any two given points in a region \cal R is that in \cal R

    \displaystyle \frac{\partial A_1}{\partial y} = \frac{\partial A_2}{\partial x},\ \frac{\partial A_3}{\partial x} = \frac{\partial A_1}{\partial z},\ \frac{\partial A_2}{\partial z} = \frac{\partial A_3}{\partial y} \cdots(26)

    where it is supposed that these partial derivatives are continuous in \cal R.

The results can be expressed concisely in terms of vectors. If \bold{A} = A_1\bold{i} + A_2\bold{j} + A_3\bold{k} , the line integral can be written \displaystyle \int_C \bold{A}\cdot d\bold{r} and condition (26) is equivalent to the condition \nabla \times \bold{A} = 0. If \bold{A} represents a force field \bold{F} which acts on an object, the result is equivalent to the statement that the work done in moving the object from one point to another is independent of the path joining the two points if and only if \nabla \times \bold{A} = 0. Such a force field is often called conservative.

The condition (26) [or the equivalent condition \nabla\times\bold{A}=0] is also the condition that A_1dx + A_2dy + A_3dz [or \bold{A}\cdot\bold{r}] is an exact differential, i.e. that there exists a function \phi(x, y, z) such that A_1dx + A_2dy + A_3dz =d\phi. In such case if the endpoints of curve C are (x_1, y_1, z_1) and (x_2, y_2, z_2), the value of the line integral is given by

\displaystyle \int_{(x_1, y_1, z_1)}^{(x_2, y_2, z_2)}\bold{A}\cdot\bold{r} = \int_{(x_1, y_1, z_1)}^{(x_2, y_2, z_2)}d\phi = \phi(x_2, y_2, z_2)- \phi(x_1, y_1, z_1)\cdots(27)

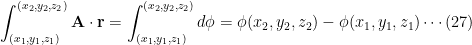
In particular if C is closed and \nabla\times\bold{A} = 0, we have

\displaystyle \oint_C \bold{A}\cdot d\bold{r} = 0 \cdots(28)

線積分が経路独立であるための条件

  • 定理 6-1.

    • 　\displaystyle \int_C [Pdx + Qdy] が領域 \cal R において与えられた任意の2点を結ぶ C から経路独立であるための必要十分条件は \cal R において

    \partial P/\partial y = \partial Q/\partial x\cdots (23)

    ここで \cal R における偏微分は連続と考えられます．

　条件 (23) はまた Pdx + Qdy が全微分であることの条件でもあります．すなわち， Pdx + Qdy = d\phi のような形をした関数 \phi(x, y) が存在することです．そのような場合，曲線 C の末端を (x_1, y_1) および (x_2, y_2) とすると，積分値は以下により得られます．

\displaystyle \int_{(x_1, y_1)}^{(x_2, y_2)}[Pdx + Qdy] = \int_{(x_1, y_1)}^{(x_2, y_2)} d\phi = \phi(x_2, y_2) - \phi(x_1, y_1) \cdots(24)

　特に，仮に (23) を満たし C が閉曲線であるなら以下により x_1 = x_2,\ y_1 = y_2 が得られます．

\displaystyle \oint_C [Pdx + Qdy] = 0\cdots(25)

　定理 6-1 の結果は空間における線積分にも応用できます．ゆえに以下が得られます．

  • 定理 6-2.

    • 　\displaystyle \int_C [A_1dx + A_2dy + A_3dz] が領域 \cal R において与えられた任意の2点を結ぶ C から経路独立であるための必要十分条件は \cal R において

    \displaystyle \frac{\partial A_1}{\partial y} = \frac{\partial A_2}{\partial x},\ \frac{\partial A_3}{\partial x} = \frac{\partial A_1}{\partial z},\ \frac{\partial A_2}{\partial z} = \frac{\partial A_3}{\partial y} \cdots(26)

    ここで \cal R における偏微分は連続と考えられます．

　その結果はベクトルの観点から簡潔に表現できます．\bold{A} = A_1\bold{i} + A_2\bold{j} + A_3\bold{k} とすると，線積分は \displaystyle \int_C \bold{A}\cdot d\bold{r} と記述でき，条件 (26) は条件 \nabla \times \bold{A} = 0 と等価です．仮に \bold{A} が物体に作用する力場 \bold{F} を表すとすると，その結果は物体をある点から他の点に移動するのになされた仕事の記述と等価であり， \nabla \times \bold{A} = 0 の時にのみ2点を結ぶ経路独立です．そのような力場のことを 保存力場 と呼びます．

　条件 (26) （または条件 \nabla\times\bold{A}=0 と等価）は又 A_1dx + A_2dy + A_3dz （又は \bold{A}\cdot\bold{r} ）が全微分であるという条件でもあります．例えば A_1dx + A_2dy + A_3dz =d\phi のような関数 \phi(x, y, z) が存在します．そのような場合では仮に曲線 C の末端を (x_1, y_1, z_1) および (x_2, y_2, z_2) とすると，線積分の値は以下により得られます．

\displaystyle \int_{(x_1, y_1, z_1)}^{(x_2, y_2, z_2)}\bold{A}\cdot\bold{r} = \int_{(x_1, y_1, z_1)}^{(x_2, y_2, z_2)}d\phi = \phi(x_2, y_2, z_2)- \phi(x_1, y_1, z_1)\cdots(27)

　特に C が閉曲線で \nabla\times\bold{A} = 0 とすると

\displaystyle \oint_C \bold{A}\cdot d\bold{r} = 0 \cdots(28)