Derivatives

このエントリーをはてなブックマークに追加
Pocket

Contents hide
1 Derivatives
2 Differentiation formulas

Derivatives

The derivative of y = f(x) at a point x is defined as

\displaystyle f'(x) = \lim\limits_{h \rightarrow 0}\frac{f(x+h) - f(x)}{h} = \lim\limits_{\Delta x \rightarrow 0}\frac{\Delta y}{\Delta x} = \frac{dy}{dx}

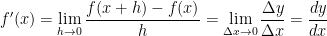
where h = Δx, Δy = f(x + h) – f(x) = f(x + Δx) – f(x) provided the limit exists.

Differentiation formulas

In the following u, v represent function of x while a, c, p represent constants. It’s assumed that the derivatives of u and v exist, i.e. u and v are differentiable.

\displaystyle \frac{d}{dx}(u \pm v) = \frac{du}{dx} \pm \frac{dv}{dx}\\\vspace{0.2 in} \frac{d}{dx}(cu) = c\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}(uv) = u\frac{dv}{dx} + v\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\left(\frac{u}{v}\right) = \frac{v(du/dx) - u(dv/dx)}{v^2}\\\vspace{0.2 in} \frac{d}{dx}u^p = pu^{p-1}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}(a^u) = a^u\ln{a}\\\vspace{0.2 in} \frac{d}{dx}e^u = e^u\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\ln{u} = \frac{1}{u}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\sin{u} = \cos{u}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\cos{u} = -\sin{u}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\tan{u} = \sec^2{u}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\sin^{-1}u = \frac{1}{\sqrt{1 - u^2}}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\cos^{-1}u = \frac{-1}{\sqrt{1 - u^2}}\frac{du}{dx}\\\vspace{0.2 in} \frac{d}{dx}\tan^{-1}u = \frac{1}{\sqrt{1 + u^2}}\frac{du}{dx}

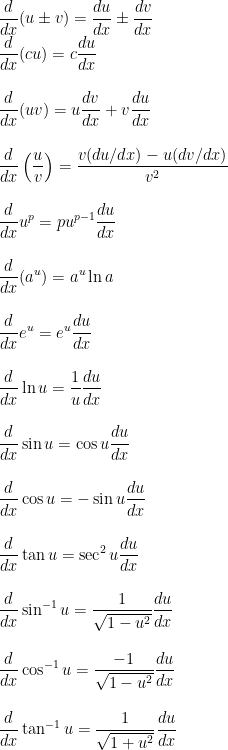
In the special case where u = x, the above formulas are simplified since in such case du/dx = 1.

このエントリーをはてなブックマークに追加
Pocket

投稿者: admin

趣味：写真撮影とデータベース． カメラ：TOYO FIELD, Hasselblad 500C/M, Leica M6． SQL Server 2008 R2, MySQL, Microsoft Access．

コメントを残す

メールアドレスが公開されることはありません。 ※ が付いている欄は必須項目です