Special first order equations and solutions

Any first order differential equation can be put into the form
$\displaystyle \frac{dy}{dx} = f(x,y)$
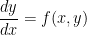
or
$\displaystyle M(x,y)dx + N(x,y)dy = 0$
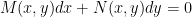
and the general solution of such an equation contains one arbitrary constant. Many special devices are available for finding general solutions of various types of first order differential equations. In the following list some of types are given.

Separation of variables

Exact equation

Integrating factor

Linear equation

Homogeneous equation

Bernoulli’s equation

Equation solvable for y

Clairaut’s equation

Miscellaneous equations

Contents hide

1 1. Separation of variables
2 2. Exact equation
3 3. Integrating factor
4 4. Linear equation
5 5. Homogeneous equation
6 6. Bernoulli’s equation
7 7. Equation solvable for y
8 8. Clairaut’s equation
9 9. Miscellaneous equations

1. Separation of variables

If differential equation is given as below,
$\displaystyle f_1(x)g_1(y)dx + f_2(x)g_2(y)dy = 0$
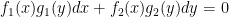
divide by $g_1(y)f_2(x) \ne 0$ and integrate to obtain general solution
$\displaystyle \int\frac{f_1(x)}{f_2(x)}dx + \int\frac{g_2(y)}{g_1(y)}dy = c$
2. Exact equation

If differential equation is given as below,
$\displaystyle M(x, y)dx + N(x, y)dy = 0$
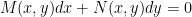
where $\displaystyle \frac{\partial M}{\partial y} = \frac{\partial N}{\partial x}$

The equation can be written as
$\displaystyle Mdx + Ndy = dU(x, y) = 0$
where dU is an exact differential. Thus the solution is $U(x, y) = c$ or equivalently
$\displaystyle \int M\partial x + \int\left(N - \frac{\partial}{\partial y}\int M\partial x\right)dy = c$
where δx indicates that the integration is to be performed with respect to x keeping y constant.

3. Integrating factor

If differential equation is given as below,
$\displaystyle M(x, y)dx + N(x, y)dy = 0$
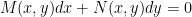
where
$\displaystyle \frac{\partial M}{\partial y} \neq \frac{\partial N}{\partial x}$
The equation can be written as an exact differential equation
$\displaystyle \mu M dx + \mu N dy = 0$
where μ is an appropriate integrating factor.

The following combination are often useful in finding integration factors.

$\displaystyle \frac{xdy - ydx}{x^2} = d\left(\frac{y}{x}\right)$
$\displaystyle \frac{xdy - ydx}{y^2} = -d\left(\frac{x}{y}\right)$
$\displaystyle \frac{xdy - ydx}{x^2 + y^2} = d\left(\tan^{-1}\frac{y}{x}\right)$
$\displaystyle \frac{xdy - ydx}{x^2 - y^2} = \frac{1}{2}d\left(\ln\frac{x - y}{x + y}\right)$
$\displaystyle \frac{xdx + ydy}{x^2 + y^2} = \frac{1}{2}d\{\ln(x^2 + y^2)\}$

4. Linear equation

If differential equation is given as below,
$\displaystyle \frac{dy}{dx} + P(x)y = Q(x)$
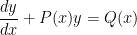
An integrating factor is given by
$\displaystyle \mu = e^{\int P(x)dx}$
and the equation can then be written
$\displaystyle \frac{d}{dx}(\mu y) = \mu Q$
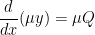
with solution
$\displaystyle \mu y = \int \mu Qdx + c$
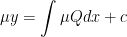
or
$\displaystyle ye^{\int Pdx} = \int Qe^{\int Pdx}dx + c$
5. Homogeneous equation

If differential equation is given as below,
$\displaystyle \frac{dy}{dx} = F\left(\frac{y}{x}\right)$
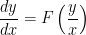
Let $y/x = v$ or $y = vx$ , and the equation becomes
$\displaystyle v + x\frac{dv}{dx} + F(x)$
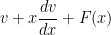
or
$\displaystyle xdv + (F(x) - v)dx = 0$
which is of Type 1 and has the solution
$\displaystyle \ln x = \int \frac{dv}{F(v) - v} + c$
where $v = y/x$ . If $F(v) = v$ , the solution is $y = cx$ .

6. Bernoulli’s equation

If differential equation is given as below,
$\displaystyle \frac{dy}{dx} + P(x)y = Q(x)y^n,\ n \neq 0, 1$
Letting $v = y^{1 - n}$ , the equation reduces to Type 4 with solution
$\displaystyle ve^{(1-n)\int Pdx} = (1 - n)\int Qe^{(1-n)\int Pdx}dx + c$
If n = 0, the equation is of Type 4. If n = 1, it is of Type 1.

7. Equation solvable for y

If differential equation is given as below,
$\displaystyle y = g(x, y)$
where
$\displaystyle p = y'$
Differentiate both sides of the equation with respect to x to obtain
$\displaystyle \frac{dy}{dx} = \frac{dg}{dx} = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}$
or
$\displaystyle p = \frac{\partial g}{\partial x} + \frac{\partial g}{\partial p}\frac{\partial p}{\partial x}$
Then solve this last equation to obtain $G(x, p, c) = 0$ . The required solution is obtained by eliminating p between $G(x, p, c) = 0$ and $y = g(x, p)$ .

An analogous method exists if the equation is solvable for x.

8. Clairaut’s equation

If differential equation is given as below,
$\displaystyle y = px + F(p)$
where
$\displaystyle p = y'$
The equation is of Type 7 and has solution
$\displaystyle y = cx + F(c)$
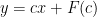
The equation will also have a singular solution in general.

9. Miscellaneous equations

If differential equation is given as below,
$\displaystyle (a) \frac{dy}{dx} = F(\alpha x + \beta y)\\\vspace{0.2 in} (b) \frac{dy}{dx} = F\left(\frac{\alpha_1 x + \beta_1 y + \gamma_1}{\alpha_2 x + \beta_2 y + \gamma_2}\right)$
(a)Letting $\alpha x + \beta y = v$ , the equation reduces Type 1.

(b)Let $x = X +h,\ y = Y + k$ and choose constants h and k so that the equation reduces to Type 5. This is possible if and only if $\alpha_1/\alpha_2 \neq \beta_1/\beta_2$ . If $\alpha_1/\alpha_2 = \beta_1/\beta_2$ , the equation reduces to Type 9(a).