Determinants | Improve Society

If the matrix $A$ in (1) is a square matrix, then we associate with $A$ a number denoted by
$\displaystyle \Delta = \left| \begin{array}{cccc} a_{11} & a_{12} & \cdots & a_{an} \\ a_{21} & a_{22} & \cdots & a_{2n} \\ \cdots & \cdots & \cdots & \cdots \\ a_{n1} & a_{n2} & \cdots & a_{nn} \end{array} \right|\cdots (9)$
called the determinant of $A$ of order n, written det(A). In order to define the value of a determinant, we introduce the following concepts.

Contents hide

1 1. Minor
2 2. Cofactor

1. Minor

Given any element $a_{jk}$ of $\Delta$ we associate a new determinant of order (n – 1) obtained by removing all elements of the jth row and kth column called the minor of $a_{jk}$ .

2. Cofactor

If we multiply the minor of $a_{jk}$ by $(-1)^{j+k}$ , the result of the elements in any row [or column] by their corresponding cofactors and is called the Laplace expansion. In symbols,
$\displaystyle \det{A} = \sum^{n}_{k=1}a_{jk}A_{jk} \cdots (10)$
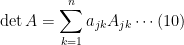
We can show that this value is independent of the row [or column] used.