Let
be an
matrix and
a column vector. The equation

where
is a number can be written as
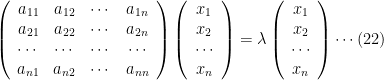
or

The equation (23) will have non-trivial solution if and only if

which is a polynomial equation of degree n in
. The roots of this equation are called eigenvalues or characteristic values of the matrix
. Corresponding to each eigenvalue there will be a solution
, i.e. a non-trivial solution, which is called an eigenvector or characteristic vector belonging to the eigenvalue. The equation (24) can also be written
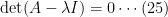
and the equation in
is often called the characteristic equation.
Eigenvalues and eigenvectors

