DOUBLE INTEGRALS | Improve Society

Let $F(x, y)$ be defined in a closed region $\cal R$ of the $xy$ plane. Subdivided $\cal R$ into $n$ subregions $\Delta\cal R$ of area $\Delta A_k,\ k = 1,\ 2,\ \dots,\ n$ . Let $(\xi_k, \eta_k)$ be some point of $\Delta\cal R$ . Form the sum
$\displaystyle \sum_{k=1}^{n}F(\xi_k, \eta_k)\Delta A_k\cdots(1)$
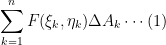
Consider
$\displaystyle \lim\limits_{n\rightarrow\infty}\sum^{n}_{k=1}F(\xi_k, \eta_k)\Delta A_k\cdots(2)$
where the limit is taken so that the number n of subdivisions increases without limit and such that the largest linear dimension of each $\Delta \cal R$ approaches zero. If this limit exists it is denoted by
$\displaystyle \iint_{\cal R}F(x, y)dA\cdots(3)$
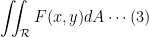
and is called the double integral of F(x, y) over the region $\cal R$ .

It can be proved that the limit dose exist if $F(x, y)$ is continuous (or piecewise continuous) in $\cal R$ .